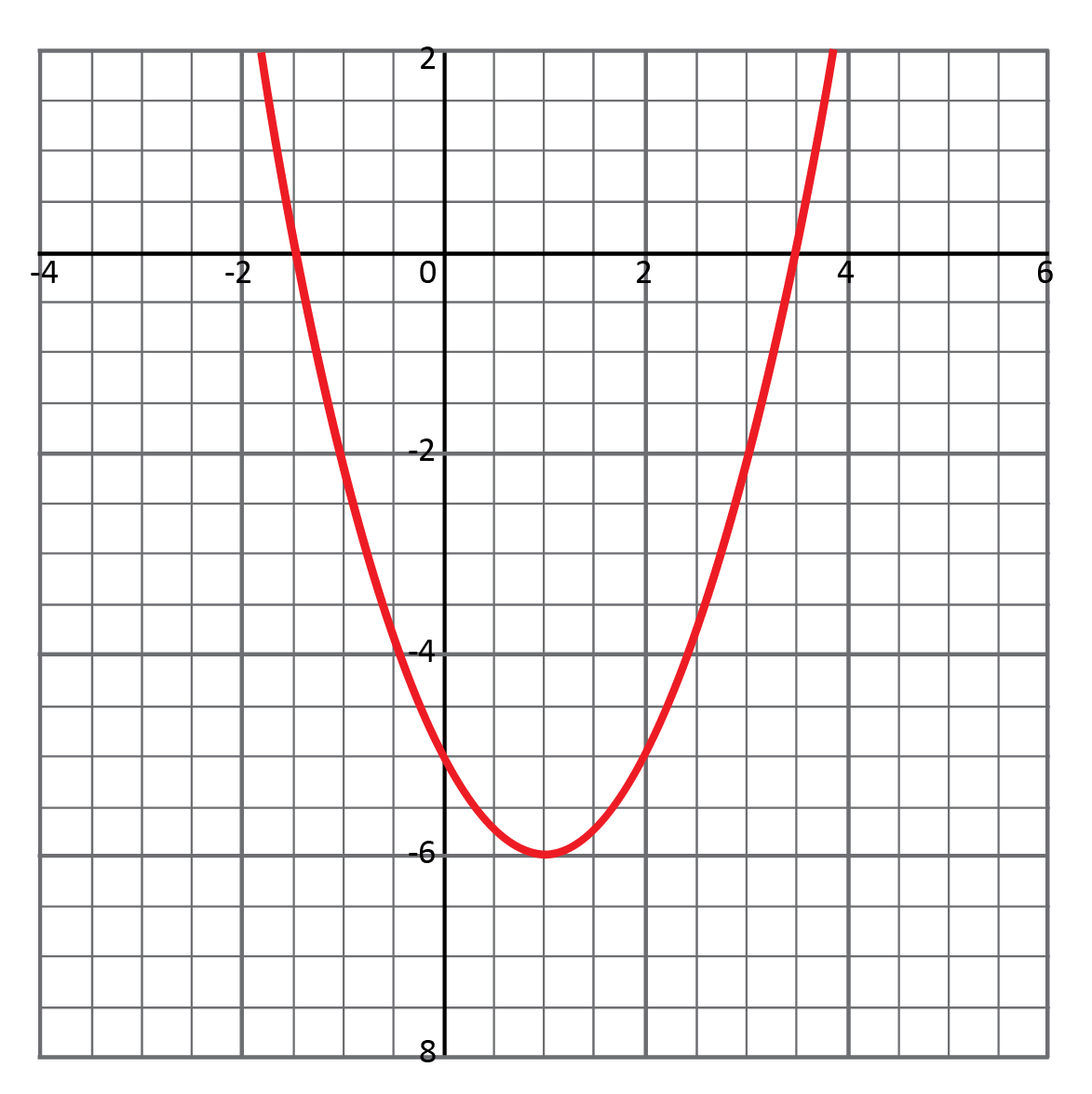
A parabola is the distinct shape of a quadratic equation when it is graphed.
In this video, we’ll give you some clues found in quadratic equations that can tell you more about the parabolas they graph.
Symmetry
So, the symmetry of a parabola is due to the squared term in the quadratic equation. However, there is one point on the parabola that does not have a “mirror image.” This point is called the vertex, and is notated by the ordered pair
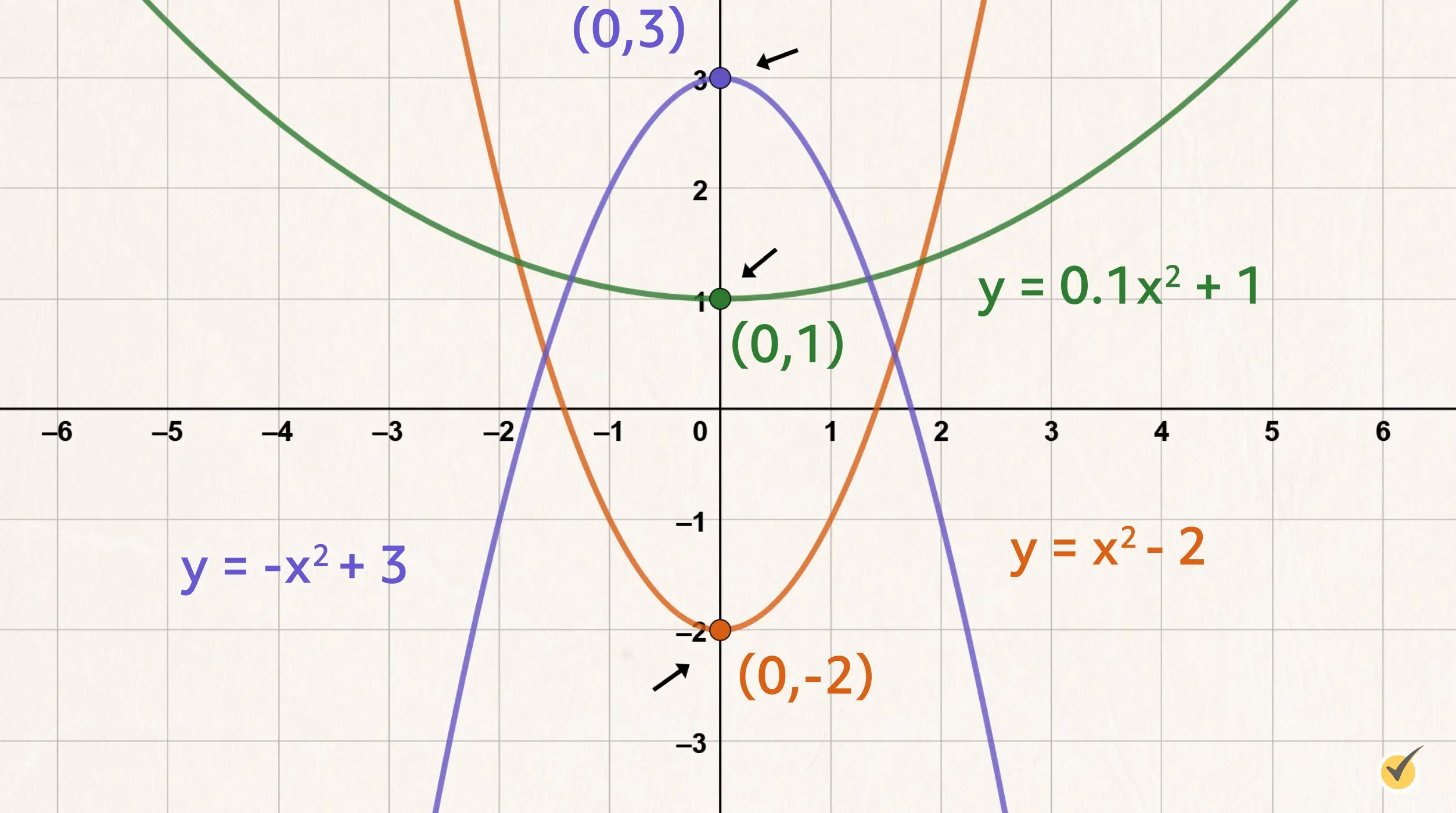
When the

However, when the
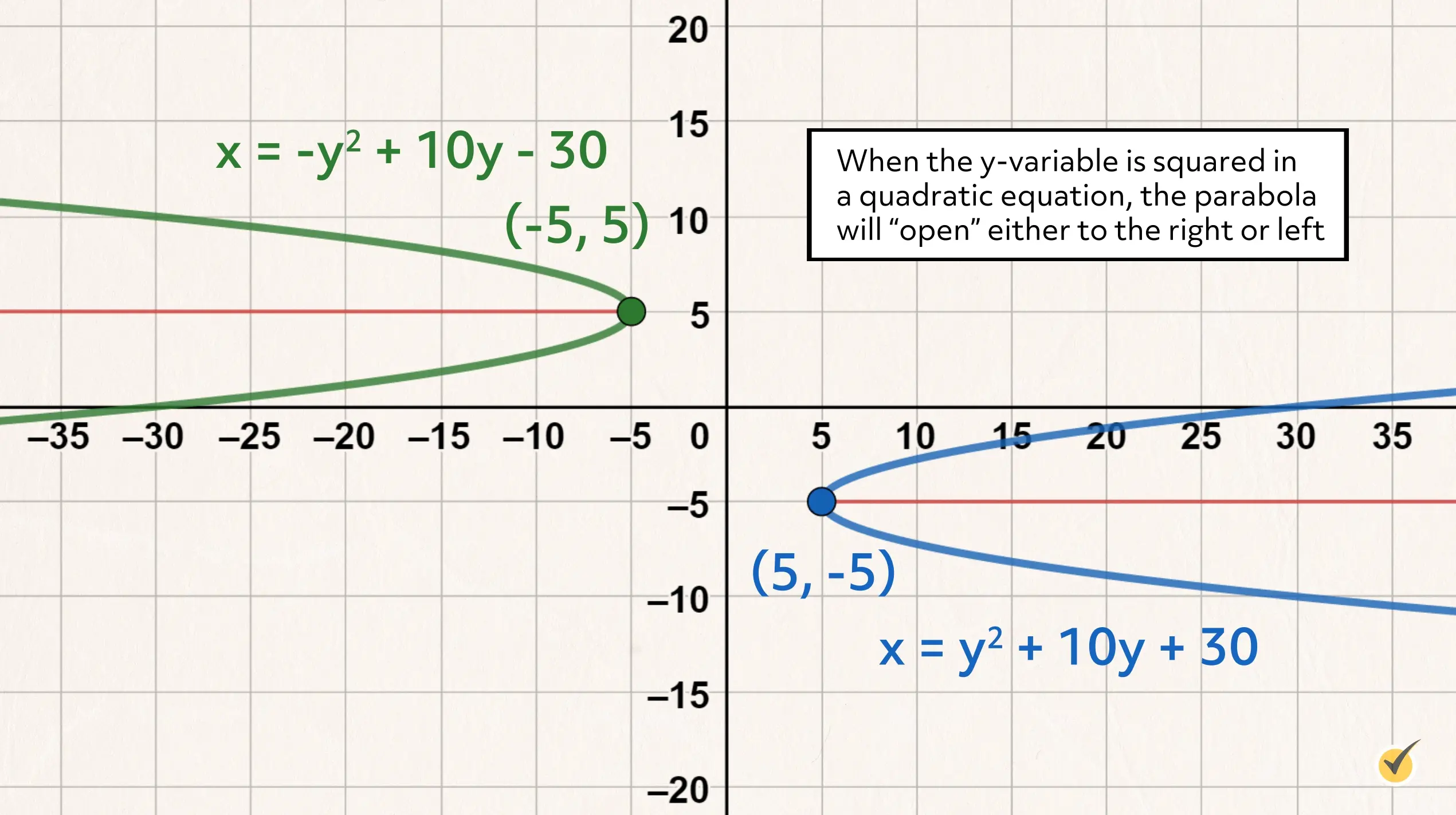
Writing Quadratic Equations
To understand this further, we need to look at how quadratic equations are written. They are written using two basic forms:
- 1. A standard form quadratic equation is written as
A quadratic equation in this form with the
- 2. A quadratic that is in vertex form is written as
As you can see, the
If the
If the
Quadratic Equation Examples
To put all of this information together, let’s look at a few examples of quadratic equations:
- 1.
This is a standard form quadratic equation with the
- 2.
This is a vertex form quadratic equation with the
- 3.
This is a standard form quadratic equation with the
- 4.
This is a vertex form quadratic with the
Thanks for watching this review of parabolas! I hope it was helpful. See you next time!
Parabola Practice Questions
What is the vertex of the parabola
What is the vertex of the parabola
Which direction does the graph of the parabola
Which direction does the graph of the parabola
What is the vertex of the parabola