Multiplying and Dividing Fractions
Many students have a real fear of fractions. However, if you can remember what a fraction represents and a few mathematical rules on how to work with them algebraically, you will be able to face fractions with confidence. In this video, we will review how to multiply and divide fractions. Let’s get started.
We should start by defining exactly what a fraction is. A fraction represents a ratio of a “part” to a “whole,” or part over whole. The value above the division line is referred to as the numerator, and the value below the division line is the denominator.
Multiplying Fractions
To multiply fractions, simply multiply “straight across,” meaning the “numerator times the numerator” divided by the “denominator times the denominator.” Let’s look at a couple of quick examples:
\(\frac{2}{3} \times \frac{2}{5}\)
Here, we want to multiply \(\frac{2}{3}\) by \(\frac{2}{5}\). As we said earlier, we’re going to multiply straight across. So we’re going to have 2 times 2 over 3 times 5. Which is equal to four over fifteen. So our answer is \(\frac{4}{15}\).
\(\frac{2}{3} \times \frac{2}{5}= \frac{2 \times 2}{3 \times 5}= \frac{4}{15}\)
Now let’s try another one. We’re going to try \(\frac{4}{7}\) times \(\frac{3}{11}\).
\(\frac{4}{7} \times \frac{3}{11}\)
Again it’s the same concept. We’re going to multiply 4 times 3, divided by 7 times 11. Which gives us \(\frac{12}{77}\).
\(\frac{4}{7} \times \frac{3}{11}= \frac{4 \times 3}{7 \times 11}= \frac{12}{77}\)
Pretty simple, right? Now let’s take a look at dividing fractions.
Dividing Fractions
Dividing fractions involves a slightly different process. Before we jump into the mechanics of the process, let’s start by looking at an intuitive example of dividing a fraction by two. The effect of dividing by 2 is simply cutting the fraction in half, or simply multiplying the fraction by 1 over 2.
So, \(\frac{4}{5}\) divided by 2 is really the same as saying \(\frac{4}{5}\) times \(\frac{1}{2}\).
\(\frac{4}{5}\)\( \div \text{ } 2 \text{ }\)\( = \frac{4}{5} \times \frac{1}{2}\)
Then it’s going to be multiplied across just like we did before. So we have 4 times 1 is four, over 5 times 2 is 10. Which then simplifies to \(\frac{2}{5}\).
\(\frac{4}{5}\)\( \div \text{ } 2 \text{ }\)\( = \frac{4}{5} \times \frac{1}{2}= \frac{4}{10}= \frac{2}{5}\)
So in other words, \(\frac{2}{5}\) is half the size of \(\frac{4}{5}\).
Similarly, dividing a fraction by 3 would result in a fraction that is one-third the size of the original:
\(\frac{2}{5}\)\( \div \text{ } 3 \text{ }\)\( = \frac{2}{5} \times \frac{1}{3}= \frac{2}{15}\)
\(\frac{2}{5}\) divided by 3 is the same as saying \(\frac{2}{5}\) times \(\frac{1}{3}\), which gives you \(\frac{2}{15}\).
So, \(\frac{2}{15}\) is one-third the size of \(\frac{2}{5}\).
Before we generalize this process, let’s review some important terminology. Consider the relationship between 2 and \(\frac{1}{2}\). These numbers are called reciprocals of one another, which means that the numerator of one number is the denominator of the other, and vice versa. Remember that 2 can be written as a fraction by writing it over 1, like this: \(\frac{2}{1}\). Therefore, \(\frac{2}{1}\) and \(\frac{1}{2}\) are reciprocals. The same is true of 3 and \(\frac{1}{3}\), because 3 can be written as \(\frac{3}{1}\). Therefore, 3 and \(\frac{1}{3}\) are reciprocals.
With this in mind, what pattern do you see in the process for dividing fractions?
Keep, Change, Flip
The process of dividing fractions is the same as multiplying the first fraction by the reciprocal of the second. A shorthand version of this wordy explanation that may help you remember the division process is “Keep, Change, Flip”:
Keep the first fraction as is
Change the operation from division to multiplication;
Flip (or take the reciprocal of) the second fraction.
Once this adjustment is made, simply follow the rules for multiplying fractions by multiplying the numerators and dividing by the product of the denominators.
Here is an example using the “Keep, Change, Flip” process:
Say we want to divide \(\frac{3}{5}\) by \(\frac{7}{5}\). We’ll keep the first fraction as is, change the operation from division to multiplication, and flip the second number. Now we just multiply our numerators, 3 times 5 is fifteen, over 5 times 7 is 35. And then from there, we simplify to \(\frac{3}{7}\).
\(\frac{3}{5} \div \frac{7}{5}= \frac{3}{5} \times \frac{5}{7}= \frac{15}{35}= \frac{3}{7}\)
I hope this video was helpful! Thanks for watching, and happy studying!
Frequently Asked Questions
Q
How do you multiply fractions with whole numbers?
A
Multiply fractions by whole numbers by turning the whole number into a fraction by placing it over 1. Any number divided by itself is itself, so this does not change the value of the whole number. Then, multiply across as with normal fractions.
Ex. \(\frac{2}{3}×4=\frac{2}{3}×\frac{4}{1}=\frac{8}{3}=2 \frac{2}{3}\)
Q
How do you multiply mixed fractions?
A
Multiply mixed fractions by first turning them into improper fractions and then multiplying across as normal. If there are common factors in the numerator and denominator, cancel those out first to simplify multiplying across. To convert the fraction back to a mixed number, divide the numerator by the denominator. The number of full divisions becomes the whole number and the remainder becomes the numerator of the fractional part over the original denominator.
Ex. \(3 \frac{1}{5}×2 \frac{7}{9}=\frac{16}{5}×\frac{25}{9}=\frac{16}{1}×\frac{5}{9}=\frac{80}{9}=8 \frac{8}{9}\)
Ex. \(3 \frac{1}{5}×2 \frac{7}{9}=\frac{16}{5}×\frac{25}{9}=\frac{16}{5}×\frac{5×5}{9}=\frac{16}{1}×\frac{5}{9}=\frac{80}{9}=8 \frac{8}{9}\)
Q
How do you divide fractions?
A
Divide fractions by using the phrase: “Keep, Change, Flip.” Keep the first fraction the same. Change the division sign to a multiplication sign. Flip the second fraction. Then multiply across and simplify if necessary.
Ex. \(\frac{4}{7}÷\frac{8}{13}=\frac{4}{7}×\frac{13}{8}=\frac{52}{56}=\frac{13}{14}\)
Q
How do you divide fractions with whole numbers?
A
Divide fractions by whole numbers by first turning the whole number into a fraction and then dividing the fractions as normal by flipping the second fraction and multiplying across. Any number can be turned into a fraction by placing it over 1.
Ex. \(\frac{2}{3}÷4=\frac{2}{3}÷\frac{4}{1}=\frac{2}{3}×\frac{1}{4}=\frac{2}{12}=\frac{1}{6}\)
Q
How do you divide mixed fractions?
A
Divide mixed fractions by first converting them to improper fractions and then dividing the fractions as normal.
Ex. \(4 \frac{3}{5}÷2 \frac{1}{2}=\frac{23}{5}÷\frac{5}{2}=\frac{23}{5}×\frac{2}{5}=\frac{46}{25}=1 \frac{21}{25}\)
Multiplying and Dividing Fractions Problems
\(\frac{2}{3}\times\frac{7}{9}=\)
The correct answer is C: \(\frac{14}{27}\). To multiply fractions, simply multiply the numerators together to get the new numerator, and multiply the denominators together to get the new denominator.
\(\frac{2}{3}\times\frac{7}{9}=\frac{2\times7}{3\times9}=\frac{14}{27}\)
\(\frac{7}{6}\div\frac{2}{3}=\)
The correct answer is A: \(\frac{7}{4}\). To divide fractions, use the phrase: Keep, Change, Flip. Keep the first fraction the same. Change the division sign to a multiplication sign. Flip the second fraction so it is its reciprocal. That process looks like this:
\(\frac{7}{6}\div\frac{2}{3}=\frac{7}{6}\times\frac{3}{2}\)
Then, multiply and simplify the fractions.
\(\frac{7}{6}\times\frac{3}{2}=\frac{7\times3}{6\times2}=\frac{21}{12}=\frac{7}{4}\)
\(\frac{1}{4}\times\frac{6}{7}\div\frac{2}{9}=\)
The correct answer is D: \(\frac{27}{28}\). According to the Order of Operations (PEMDAS), multiplication and division can happen at the same time. For this example, let’s work through the multiplication and division in order from left to right. So we’ll start by multiplying \(\frac{1}{4}\) and \(\frac{6}{7}\), simplifying if necessary.
\(\frac{1}{4}\times\frac{6}{7}=\frac{1×6}{4×7}=\frac{6}{28}=\frac{3}{14}\)
Then, divide \(\frac{3}{14}\) by \(\frac{2}{9}\).
\(\frac{3}{14}\div\frac{2}{9}=\frac{3}{14}\times\frac{9}{2}=\frac{27}{28}\)
Therefore, \(\frac{1}{4}\times\frac{6}{7}\div\frac{2}{9}=\frac{27}{28}\).
Sarah Anne is baking cookies and the recipe calls for \(\frac{2}{3}\) cups of butter. She needs a lot of cookies, so she decides to quadruple the recipe. Quickly realizing that’s a lot of cookies, she decides to back off a bit and only make the recipe \(3\frac{1}{2}\) times the original. In order to do this, how many cups of butter will Sarah Anne need?
\(2\frac{1}{3}\) cups
\(\frac{4}{21}\) cups
\(1\frac{2}{3}\) cups
\(\frac{1}{7}\) cups
The correct answer is A: \(2\frac{1}{3}\) cups. The first thing that needs to happen in order to solve this problem is \(3\frac{1}{2}\) needs to be converted to an improper fraction.
\(3\frac{1}{2}=\frac{3×2+1}{2}=\frac{7}{2}\)
Then, multiply the two fractions and simplify.
\(\frac{2}{3}\times\frac{7}{2}=\frac{14}{6}=\frac{7}{3}\)
Finally, convert \(\frac{7}{3}\) to a mixed number.
\(\frac{7}{3}=2\frac{1}{3}\)
Sarah Anne needs \(2\frac{1}{3}\) cups of butter.
Antonio has \(\frac{6}{8}\) of a pizza left over and two hungry friends. If Antonio and his friends evenly split the pizza, what fraction of the pizza does each person get?
The correct answer is C: \(\frac{1}{4}\). This question is asking us to divide \(\frac{6}{8}\) by 3. Remember, any whole number can be turned into a fraction by placing it over 1. Here’s what the division looks like:
\(\frac{6}{8}\div\frac{3}{1}=\frac{6}{8}\times\frac{1}{3}=\frac{6}{24}=\frac{1}{4}\)
Each person gets \(\frac{1}{4}\) of the pizza.
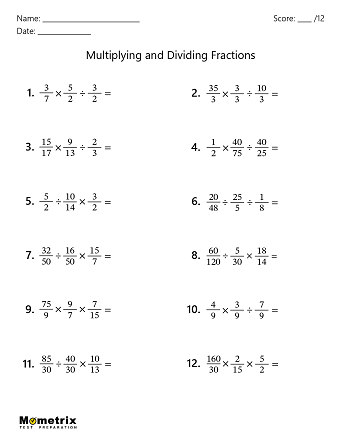
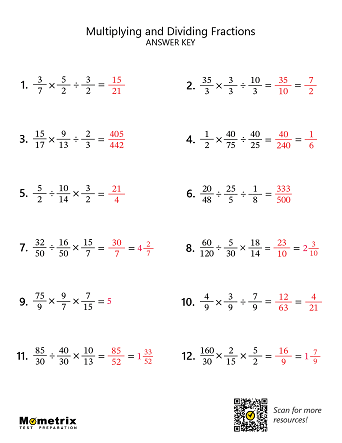
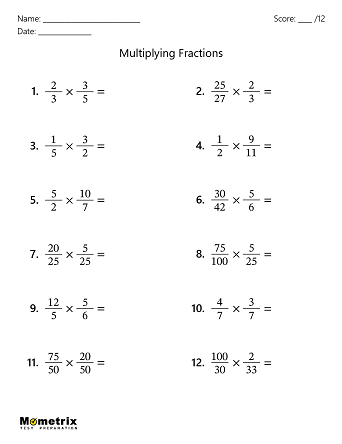
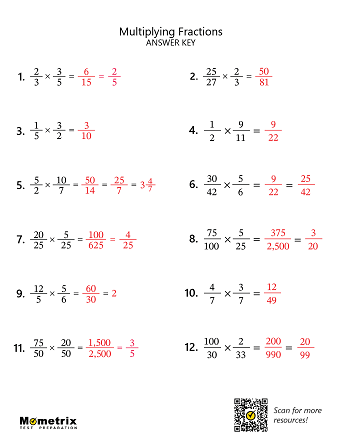
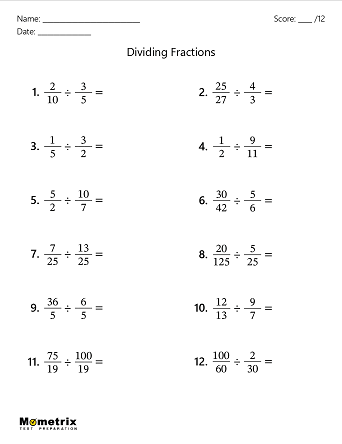
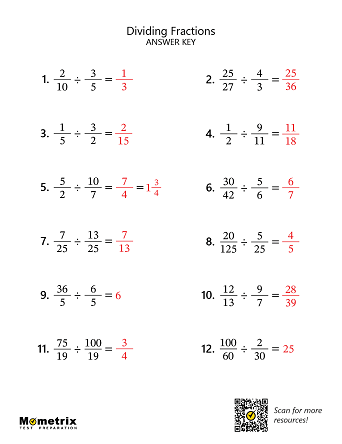
Multiplying and Dividing Fraction Worksheets
Use our free printable multiplying and dividing fractions worksheets for additional practice!
