
Hi, and welcome to this review of logarithmic functions!
Like all mathematical functions, logarithms are used to measure and model real-life occurrences. From sound measured in decibels to the magnitude of earthquakes measured on the Richter Scale, logarithms are used to relate these natural occurrences to a baseline measurement.
In addition, logarithmic functions have an inverse relationship with exponential functions, meaning that they “undo” each other. This allows us to use logarithms as a tool to solve exponential equations.
If you want to try some of the examples in this video on your own, grab a scientific calculator and be prepared to practice some skills and explore the power and properties of logarithmic functions.
Let’s get started!
Exponential Equation Basics
Before we start our work with logarithms, let’s take some time to review the basics of exponential equations.
A base of 2 raised to the power of 3 is equal to 8. The answer, 8, is referred to as the argument. Rearranging these components allows us to write the inverse logarithm, as shown highlighted in green. This illustrates nicely that logs are the power of a named base.
Example #1
Let’s practice identifying these components and re-writing a few exponential equations as logarithmic equations:
Normally we would say, “five squared equals 25.” If we were to use the terms we used in our first example, we could say, “a base of 5 raised to the power of 2 is equal to 25.”
Now, let’s rewrite this as a logarithm:
Example #2
Let’s try another one. If you’d like to try this out on your own, pause the video and give it a shot!
We would say this is a base of 4 raised to the power of 3 is equal to 64.
If we rewrite this as a logarithm, we get this:
When we evaluate a logarithmic expression we ask ourselves, “What do I have to raise this base by to get the value of the argument?” Logarithms are the power that is needed.
Logarithm Notation
Now, let’s focus on the notation of logarithms.
In our first example, the base of the log was 5, and our second example had a base of 4. Note that the base is indicated as a subscript on the word, “log.” This will be true for logs with bases other than 10 and the irrational number, \(e\).
Logarithms with a base of 10 do not indicate the base in the notation, and they are called “common logs.” There is a key on a scientific calculator for common logs. If you have a calculator on hand, try inputting the following: \(\text{log } 1000\).
The answer is 3, which means that 3 is the exponent needed to raise a base of 10 to get to 1,000. See if you can guess the answer to this one before you see the answer: \(\text{log } 10\).
Think you got it? The answer is 1. A base of 10 raised to the power of 1 will result in 10.
Natural Log
There is also a key for the “natural” log function, which reads, “ln.” Whenever you see this notation, you know that you are dealing with a base of \(e\). Exponential functions that deal with continuously compounded interest or population growth models have a base of \(e\). The natural log is used to solve such applications, but that’s a topic for another time.
The Richter Scale
As mentioned earlier, the Richter scale measures the amplitude of waves that result from seismic activity and uses a logarithmic formula \(R=log(\frac{A}{B})\), where \(A\) is the observed amplitude and \(B\) is a baseline measure.
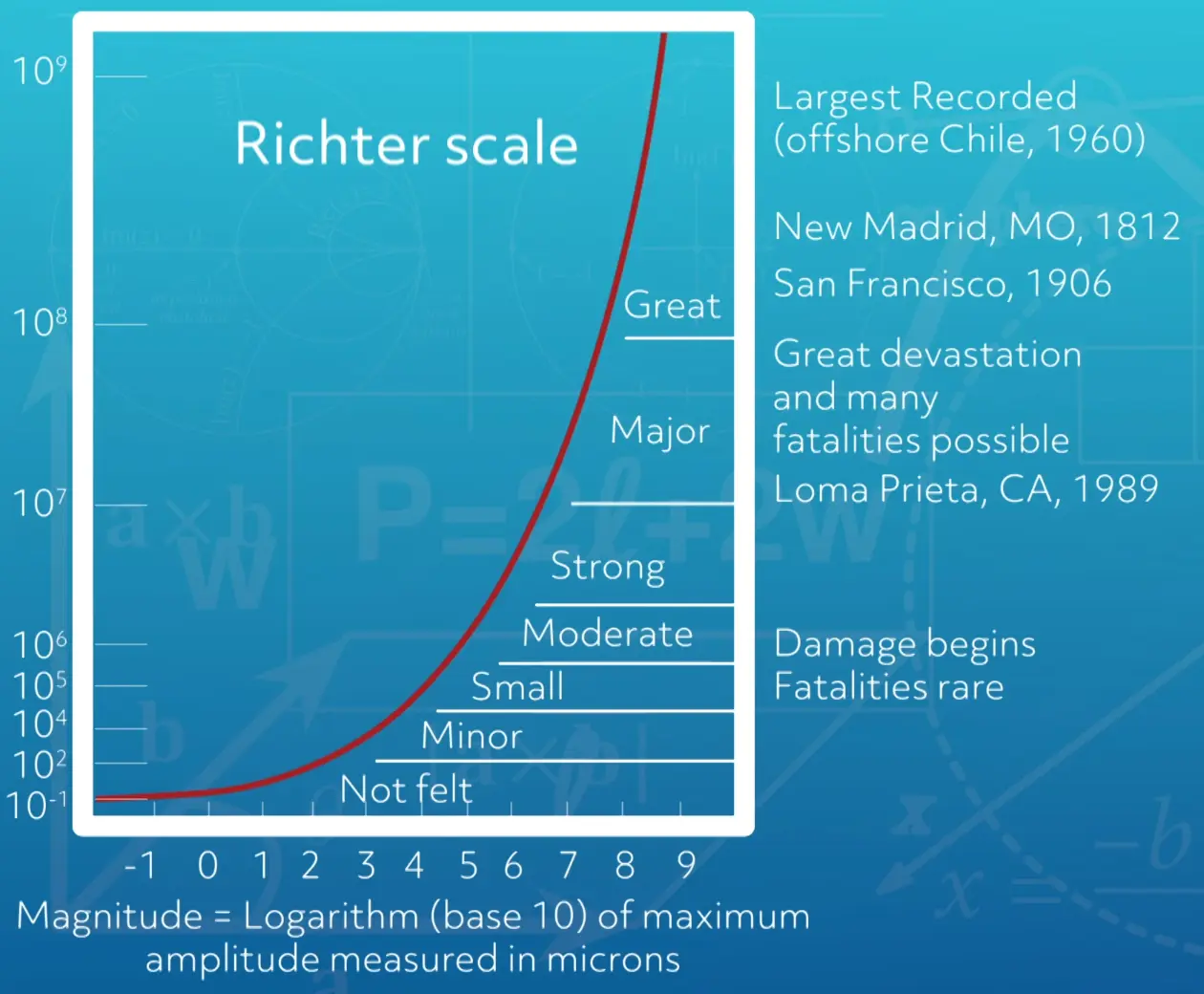
If the amplitude of a wavelength is measured at 450 times the baseline, the formula would read as \(R=\text{log }(\frac{450\times B}{B})\), which simplifies to \(R=\text{log }(450)\). Entering this in your calculator results in a value of 2.7, rounded to the nearest tenth. This measurement reflects the exponent on a base 10, and would be considered a fairly minor earthquake on the Richter Scale.
Graphing Logarithmic Functions
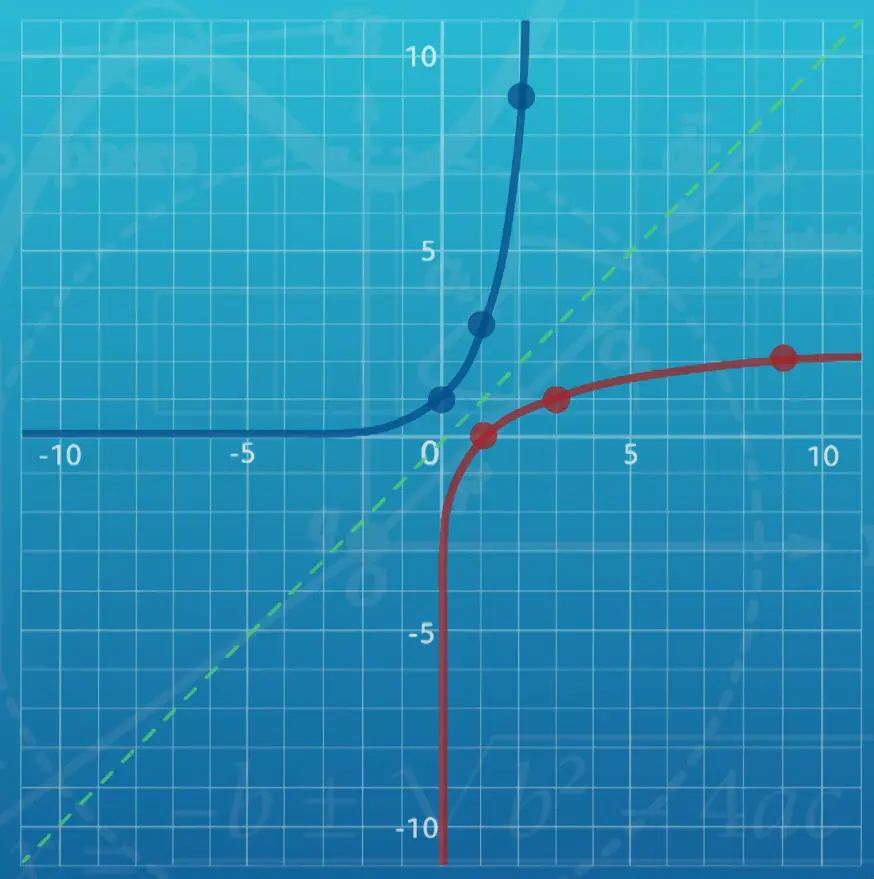
Like all inverse functions, the graphs of exponential and logarithmic functions are symmetric about the identity line \(y = x\).
Graphing a logarithmic function is as simple as creating a table and plugging in values until the curve takes shape.
Some students find it is easier to convert the logarithm to an exponential function and “flip” the domain and range values to get the mirror image graph.
Let’s give that method a try:
Graph the exponential function \(f(x) = 3^x\) and the logarithmic function \(g(x)=\text{log}_3(x)\) on the same graph.
There are several properties of logarithms that allow you to rewrite expressions in order to simplify them. I will list the corresponding exponent rule that you are already familiar with that supports the logarithmic function. Given what we have already covered with respect to exponential expressions, the first three should seem rather intuitive:
Property #1
Remember to ask yourself, “What base of \(b^0\) will give me an answer of 1?” Well, we know that any base raised to a power of 0 equals 1.
Property #2
This also makes sense, because raising any base to a power of 1 is equal to the base.
Property #3
This is similar to Property #2, but the exponent, \(n\), can be any value. Thinking about the inverse relationship with an exponential also may be helpful here: taking the log of an exponential “undoes” the exponential. The result is the input, \(n\).
The next three properties are more arithmetic and are used to simplify or expand logarithmic expressions. I will provide some examples for each of these properties.
Property #4 – The Product Property
The key with this property is that you can “expand” the log of a product to the sum of logs with the same base. This relates to the exponent rule.
Here is an example:
Write the logarithm as a sum and simplify, if possible.
For this, we’ll use Property #2 to simplify the first term of the expression on the right:
Here’s another example. Write the logarithm as a sum and simplify, if possible.
\(\text{log}_3(9) + \text{log}_3(x)\)
To simplify it, let’s rewrite \(3^2\).
Then, we’ll use Property #3 to simplify further. This gives us:
Let’s take a look at another property.
Property #5 – The Quotient Property
As you can see, the log of a quotient can be expanded into the subtraction of two logs of the same base. The associated exponent rule is \(\frac{a^m}{a^n}=a^{m-n}\).
Let’s practice expanding a logarithm using this property.
We need to be sure to keep the bases the same for the subtracted terms! The first term of the expression on the right can be simplified by writing 49 as \(7^2\).
Now, we can simplify with Property #3. This gives us:
Now let’s work on the property backwards to condense the subtraction of logs with the same base to a quotient:
Now we’re gonna create the quotient.
Simplify the quotient.
You have condensed the expression to one log!
The next property involves the exponent on the argument of a logarithm.
Property #6 – The Power Property
Note that when the argument is raised to a power, the expression is equal to the exponent being multiplied by the logarithm. The associated exponent rule is \((a^m)^n=a^{m \times n}\).
We will practice implementing this property with a more comprehensive example just to keep things interesting!
Notice that there is no subscript indicated! What is the base? Remember, if there is no subscript indicated, the base is always 10.
Now, we need to identify the Power Property in the second term. The multiplier of the 3 before the log translates to the argument of 3 being raised to the third power. So this is the same as saying,
Use the Product Rule to combine the sum of \(\text{log}(2)\) and \(\text{log}(3^3)\).
Now, use the Quotient Rule to condense the subtraction of common logs to a single common log.
Simplify the quotient by canceling out the common factor of 9, for a final answer of
For our final practice problem, let’s go the other way and expand a single log into an expression of addition and subtraction:
First, we’ll expand the quotient into subtraction of two logs, base 3.
Then, we’ll expand the product in the first term to the sum of logs, base 3.
Now, we need to identify the Power Property in the second term. Move the exponent of 2 to the front of the log as a multiplier.
Simplify the first term using Property #2.
Okay, that about wraps things up! I hope that the examples that we worked through have helped you better understand logarithms, their relationship with exponential functions, and the properties that allow us to simplify complex expressions.
Thanks for watching, and happy studying!
Logarithmic Function Practice Questions
Rewrite the exponential equation below as a logarithmic equation.
\(7^3=343\)
In a logarithmic equation, the subscript next to “log” represents the base. The portion in parentheses is called the argument. The number after the equal sign is the power of the named base. In the given exponential equation, the base is \(7\), the argument is \(343\), and the power is \(3\). Answer D correctly rearranges these components to form the equivalent logarithmic equation.
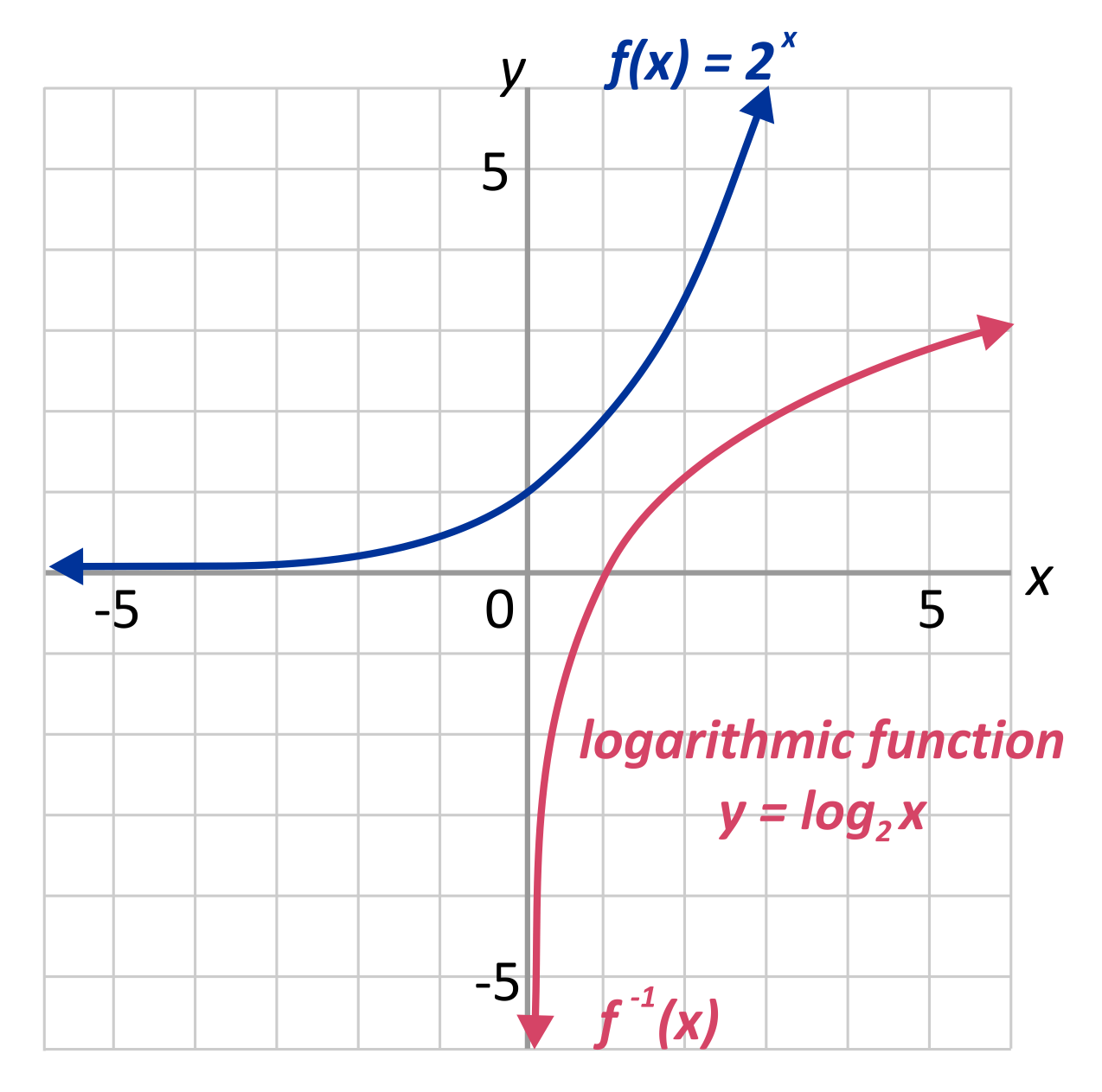
Fill in the blank: “Graphs of logarithmic functions and exponential functions are __________ in relation to the identity line, \(y=x\).”
Since logarithmic functions and exponential functions are inverses of each other, they are symmetrical about the identity line \(y=x\). This concept is illustrated in the graph below.
Mark created a table for the exponential function \(f(x)=2x\) and plugged in corresponding values. His table is shown below. Mark’s partner created a table for the logarithmic function \(g(x)=\text{log}_2x\). Based on Mark’s work, which table should his partner have created?
\(f(x)=2^x\)
| \(x\) | \(f(x)\) |
| \(0\) | \(1\) |
| \(1\) | \(2\) |
| \(2\) | \(4\) |
| \(3\) | \(8\) |
| \(x\) | \(0\) | \(1\) | \(2\) | \(3\) |
| \(g(x)\) | \(8\) | \(4\) | \(2\) | \(1\) |
| \(x\) | \(3\) | \(2\) | \(1\) | \(0\) |
| \(g(x)\) | \(1\) | \(2\) | \(4\) | \(8\) |
| \(x\) | \(1\) | \(2\) | \(4\) | \(8\) |
| \(g(x)\) | \(3\) | \(2\) | \(1\) | \(0\) |
| \(x\) | \(1\) | \(2\) | \(4\) | \(8\) |
| \(g(x)\) | \(0\) | \(1\) | \(2\) | \(3\) |
To convert a table for an exponential function into a logarithmic function, flip the domain and range values to get the mirror image graph. Since the values of x in the exponential function are \(0,1,2,\) and \(3\), the values of \(g(x)\) in the logarithmic function must also be \(0,1,2,\) and \(3\). Likewise, since the values of \(f(x)\) in the exponential function are \(1,2,4,\) and \(8\), the values of \(x\) in the logarithmic function must also be \(1,2,4,\) and \(8\). Therefore, answer D is correct.
Write the logarithm \(\text{log}_3(27)\) as a sum and simplify if possible.
| \(\text{log}_3(27)=\text{log}_3(3)+\text{log}_3(9)\) | First, use the Product Property to rewrite \(\text{log}_3(27)\) as the sum of \(\text{log}_3(3)\) and \(\text{log}_3(9)\) since \(3\times9=27\). |
| \(1+\text{log}_3(9)\) | Next, use Property 2, \(\text{log}_b(b)=1\) to rewrite \(\text{log}_3(3)\) as \(1\). |
| \(1+\text{log}_3(3^2)\) | Then, rewrite \(\text{log}_3(9)\) as \(\text{log}_3(3^2)\) since \(3^2=9\). |
| \(1+2=3\) | From here, use Property 3, \(\text{log}_b(b^n)=n\), to rewrite \(\text{log}_3(3^2)\) as \(2\). Since \(1+2=3\), the logarithm can be simplified to \(3\). Therefore, answer A is correct. |
Which property is illustrated below?
\(\text{log}_8(12)-\text{log}_8(4)\)
\(\text{log}_8(\frac{12}{4})\)
\(\text{log}_8(3)\)
In the example given, the logarithmic expression \(\text{log}_8(12)-\text{log}_8(4)\) is simplified using the Quotient Property. The Quotient Property is as follows: \(\text{log}_b(\frac{m}{n})=\text{log}_b(m)-\text{log}_b(n)\). The example gave \(\text{log}_b(m)-\text{log}_b(n)\), which needed to be simplified to \(\text{log}_b(\frac{m}{n})\). Therefore, answer C is correct.
