
When we think “even and odd,” usually even and odd numbers are what come to mind. But what are even and odd functions? In today’s video, we will define even and odd functions and discuss how to identify them.
Even Functions
Let’s begin by talking about even functions. If a function \(f(x)\) evaluated at \(-x\) gives us the same \(f(x)\) we started with, that function is even.
Formally written:
\(f(x)\) is even. Graphically, an even function will appear symmetric, or mirrored, about the vertical y-axis. One example of an even function is \(f(x)=x^{2}\).
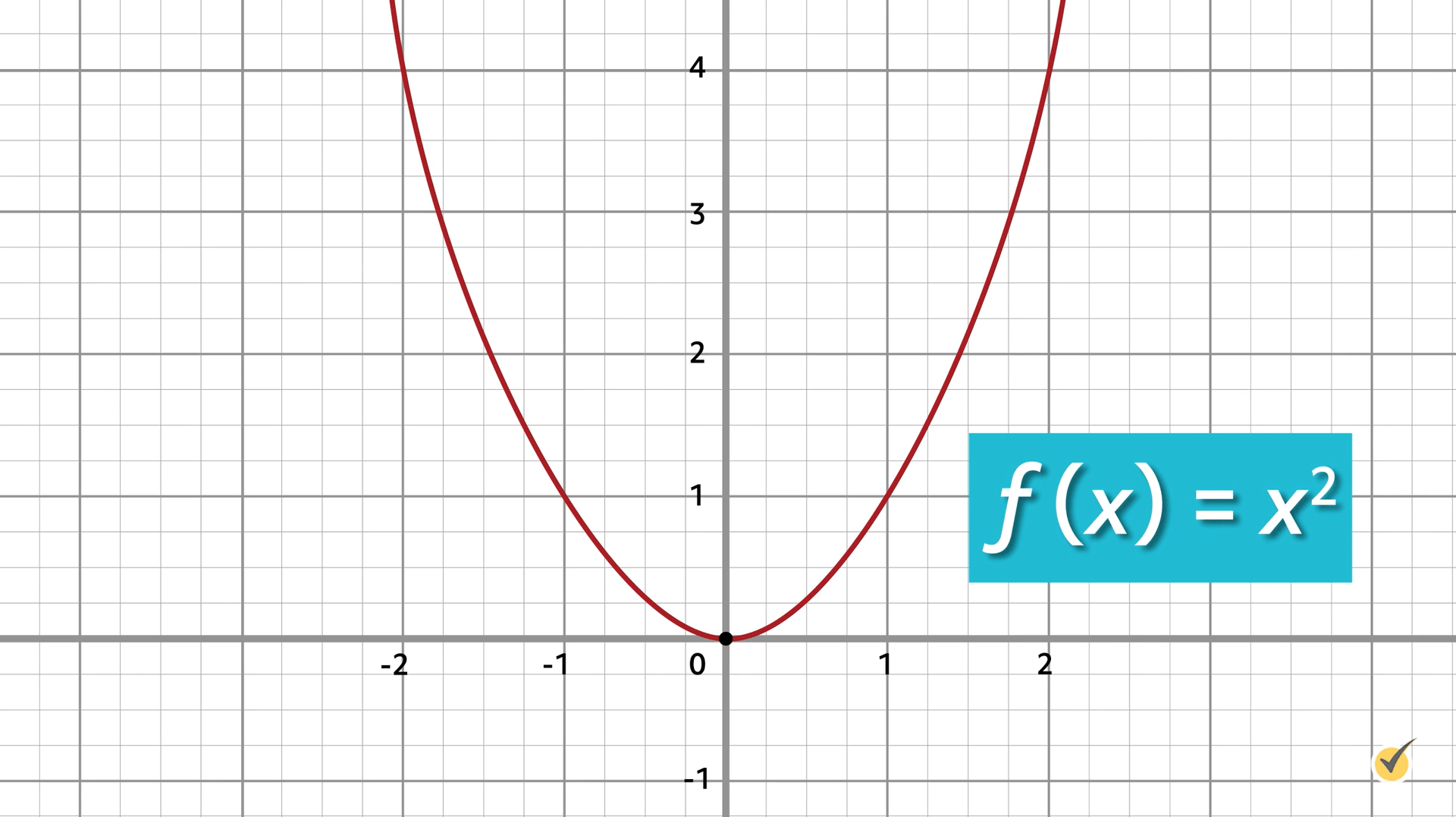
Notice that the shape of this familiar parabola is visibly symmetric. The left and right sides of the plane are identical, just flipped. We can also show that this function is even algebraically, by evaluating at \(-x\).
So our original function is \(f(x)=x^{2}\). And we said that, if \(f(-x)\) is the same as \(f(x)\), then the function is even. So let’s evaluate at \(-x\). So, wherever there’s an \(x\), we’re gonna plug in \(-x\).
So we have:
Which, when you square a negative, it turns positive, so this is equal to:
So in this case, \(f(-x)=f(x)\). And because of our definition of even, this function, \(f(x)=x^{2}\), is even.
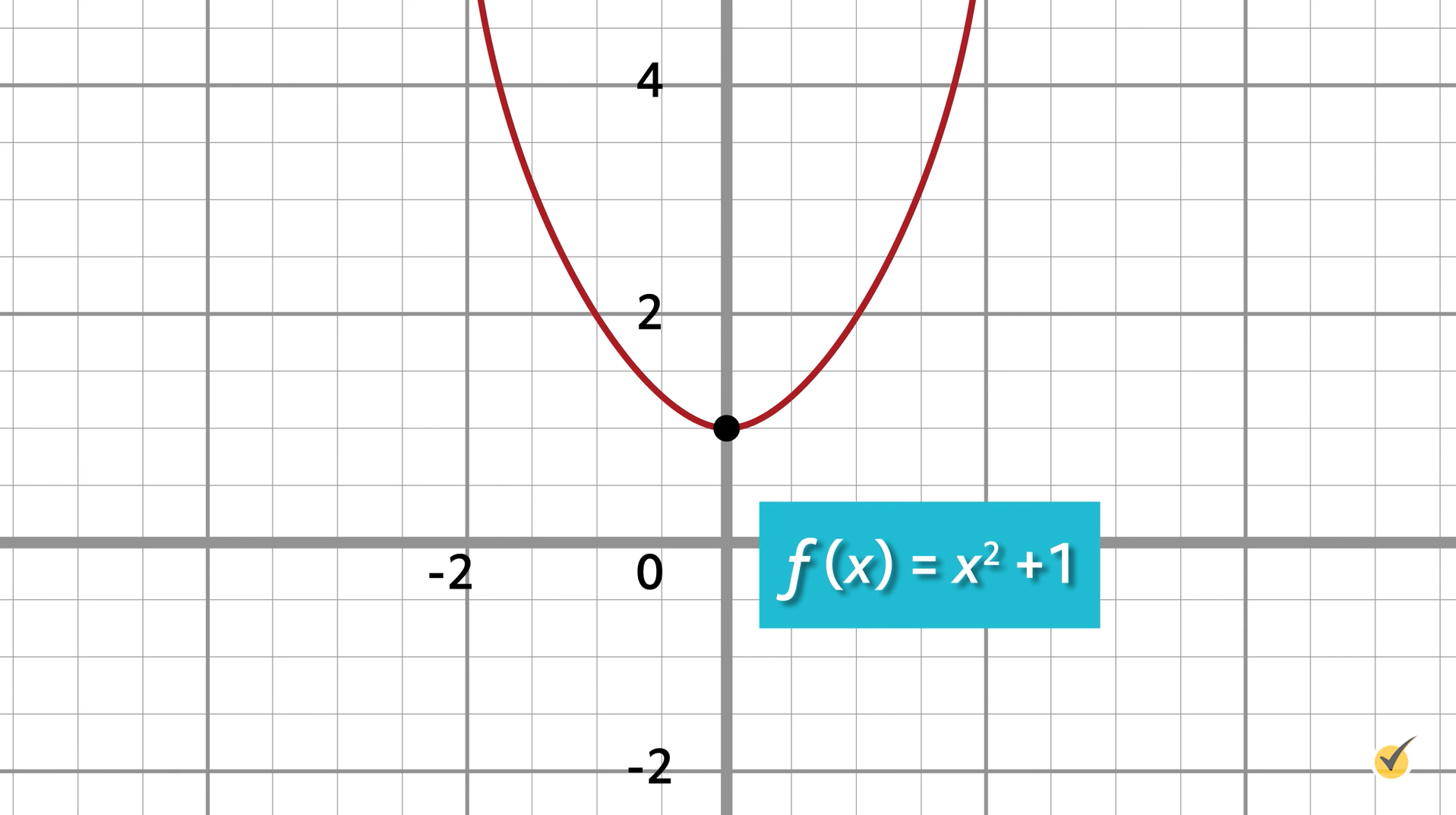
Notice that if we add a constant to this function, it won’t affect the shape of the function, just raise or lower it on the plane. For example, this is the graph of \(f(x)=x^{2}+1\). Notice how it still has the same shape, and it is still an even function. It has just been moved up one unit on the coordinate plane.
Odd Functions
Now let’s talk about what odd functions are like.
Consider another function \(f(x)\), which we will once again evaluate at \(-x\). But this time, instead of looking for the same \(f(x)\) we started with, we want to see if \(f(-x)\) changes the sign of all terms in the function.
In other words, if \(f(-x)=-f(x)\), then the function is odd.
Graphically, an odd function will appear the same when we rotate it by 180°, like flipping a page upside down, and it must pass through the origin.
A few examples of odd functions are:
\(f(x)=x^{3}\)
\(f(x)=sin(x)\)
Let’s take a look at what’s going on here algebraically, using \(f(x)=x^{3}\) as an example.
So we’re gonna have our original function: \(f(x)=x^{3}\). And just like before, we’re gonna evaluate it at \(-x\). So we want \(f(-x)\). So anywhere we see an \(x\), we’re gonna plug in \(-x\).
So \((-x)^{3}\), which is \(-x\cdot-x\cdot-x\), which means, since there are three negatives, our final answer’s gonna be \(-x^{3}\).
So if you notice, \(f(x)\) is the opposite of \(f(-x)\). Each term, which in this case we only have one, is changed from positive to negative. So that means that this function is odd.
Notice that if we were to add a constant to this function, it would no longer be odd. Remember that for odd functions, every term must change signs when evaluating at \(-x\). That constant term would have no way to change sign, and we would see on the graph that the function would no longer pass through the origin.
Functions That Are Neither Even Nor Odd
So we have now talked about definitions of both even and odd functions but before we go further, it’s important to clarify that some functions may be neither even nor odd! For example, take a look at the function \(f(x)=(x+1)^{2}\).
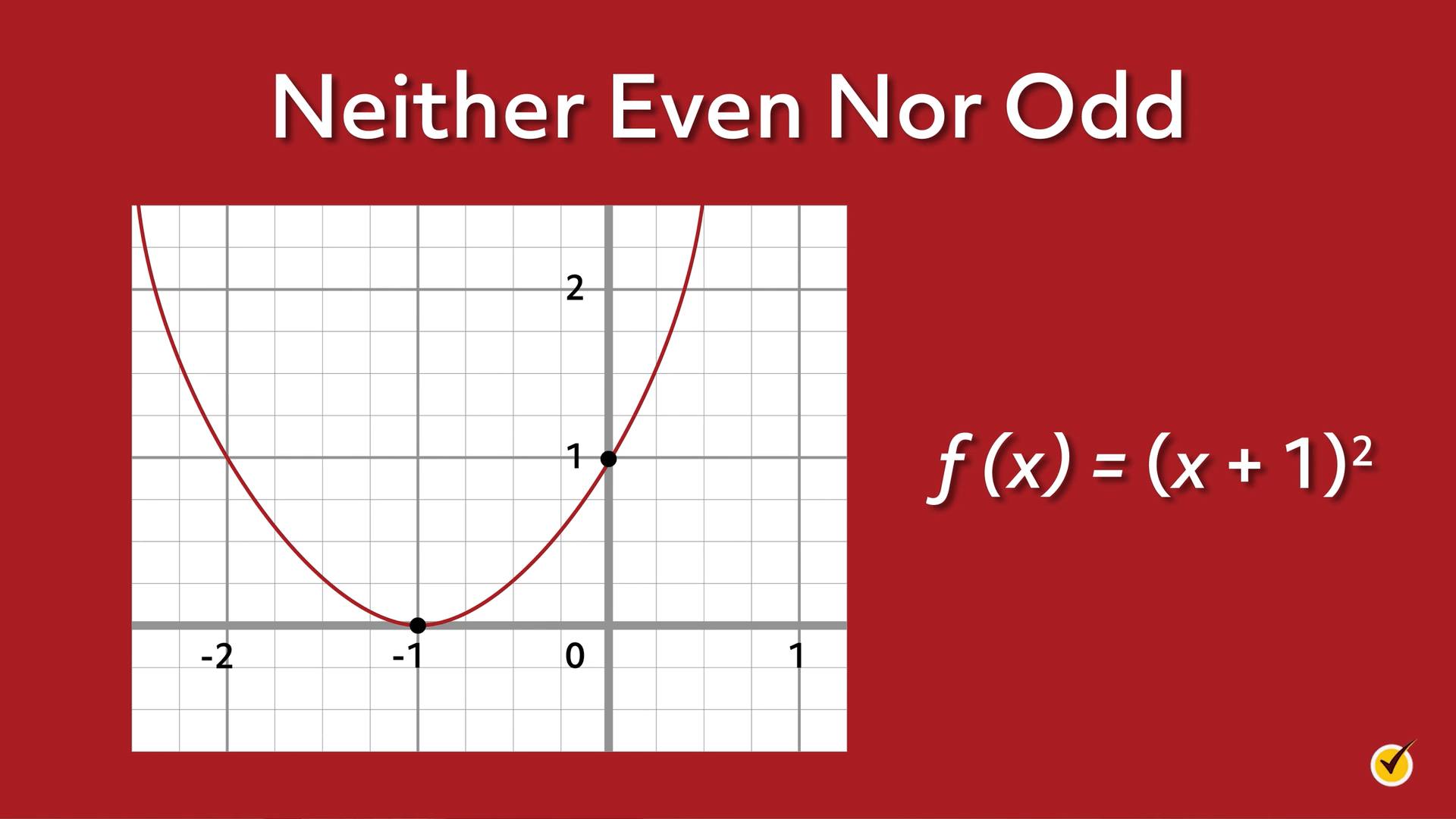
From the graph we can see that this function doesn’t pass through the origin, so it can’t be odd. And it isn’t symmetric about the \(y\)-axis, so it isn’t even either. But we can also determine this algebraically.
So our function is \(f(x)=(x+1)^{2}\). And remember, to determine if it’s even or odd, we want to evaluate it at \(-x\).
So we have:
Which we can write as:
And then from here we can FOIL:
\(1\cdot-x=-x\)
\(-x\cdot1=-x\)
So we have:
But remember, to determine even or odd, we have to compare this to our original function. So let’s expand that.
For this, we have:
Which is:
\(1\cdot x=x\text{ plus }x\cdot1=x,\)\(\text{ so plus }2x\)
\(1\cdot1=1\)
\(f(x)=(x+1)(x+1)\)\(=x^{2}+2x+1\)
Now, when we compare these two functions, we see that only one of the three terms ended up changing signs, so \(f(x)\) is not odd. And since one term did change sign, \(f(x)\neq f(-x)\), so the function is not even either.
The Reason for Even and Odd Functions
Now that we’ve laid a groundwork for understanding even and odd functions, let’s talk about why we call them even and odd in the first place.
Remember that even functions are the same when we evaluate them at \(+x\) and at \(-x\). As we saw earlier, \(f(x)=x^{2}\) satisfies this property because anytime we square something, a positive value is returned, and therefore the sign of that term doesn’t change even if we plug a negative value in. The same is true when something is raised to the fourth power, or the sixth, and so on.
Notice that constants do not change sign when we evaluate at \(-x\) either. That’s why we saw that the function \(f(x)=x^{2}+1\) was still even. As you can see, an even function will have even exponents.
It may be unsurprising now that odd functions likewise will have odd exponents! Remember that in order for a function to be odd, all terms must change sign when we evaluate at \(-x\).
Clearly, any term with \(x\) to the first power will change sign when we plug in a negative value of \(x\). In the same way, \(x\) to the third power, the fifth power, and so on will all change sign when we plug in a negative value for \(x\).
As we mentioned earlier, when a term has an even power of \(x\), it will not change sign. That means that an odd function cannot contain any terms with even powers of \(x\), and it cannot have any constants.
You may recognize even and odd functions later on in calculus when it comes to dealing with the Taylor expansion.
Review Questions
Time for some practice problems!
Question #1
Based on this graph, is this function even, odd, or neither?
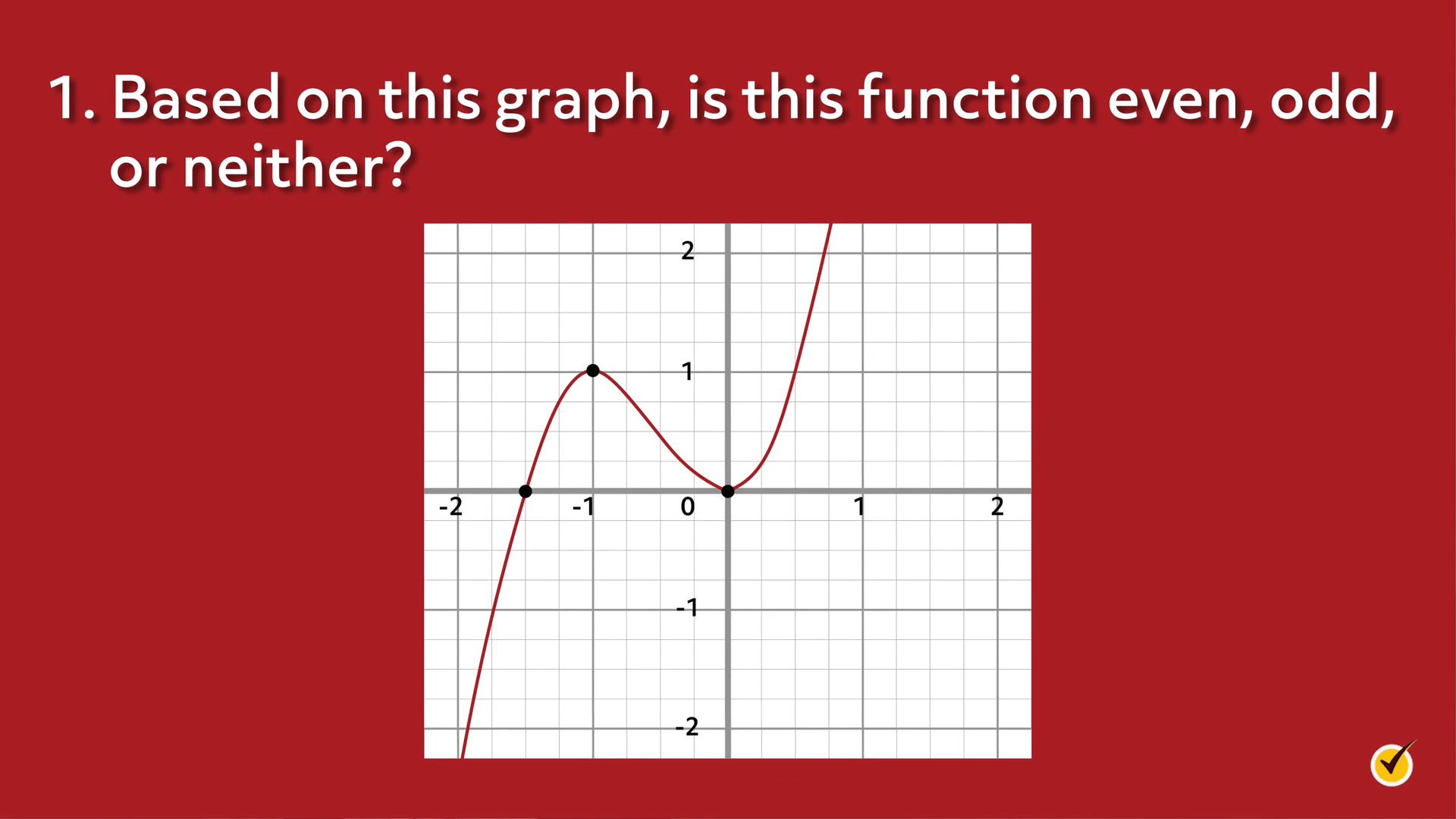
Neither. This function is not symmetric about the \(y\)-axis, so it is not even. And even though it passes through the origin, it is not odd either because it would not appear the same if we were to rotate the image 180°.
Question #2
Let’s try another one.
Is the function \(f(x)=\frac{5}{7}x^{3}-2x\) even, odd, or neither?
Let’s look at each term. First, \(\frac{5}{7}x^{3}\) has an odd power of \(x\), meaning that the sign will change when evaluated at \(-x\). Similarly, the second term, \(-2x\), has an odd power of \(x\) and will also change sign. That means this function is odd!
Question #3
Let’s finish with a more conceptual question.
We know that some functions may be neither even nor odd, but is it possible for a function to be both even and odd?
Surprisingly, the answer is yes, but only for one function. Can you think of what function that is? Remember that for even functions, \(f(-x)=f(x)\), and for odd functions, \(f(-x)=-f(x)\). The only way both of these can be satisfied is when \(f(x)=0\).
\(and\)
\(f(-x)=-f(x)\)
As a quick recap, we can identify even and odd functions in the following ways:
Graphically, even functions are symmetric about the \(y\)-axis. And they don’t have to pass through the origin. Though, odd functions must pass through the origin, and they will appear the same when viewed from a 180° rotation.
Algebraically, even functions are the same when we evaluate at \(+x\) and at \(-x\). Odd functions will change signs across all terms when evaluated at \(-x\).
As a shortcut, if a function contains only even exponents of \(x\) (and may or may not have constants) then it is even. If a function has no constants and only odd exponents of \(x\), then it is odd.
Now that we’ve covered everything and run through some examples, you should be pretty comfortable with identifying even and odd functions.
Thanks for watching, and happy studying!
Even and Odd Function Practice Questions
The graph of the function \(y=f(x)\) is shown on the coordinate plane below.
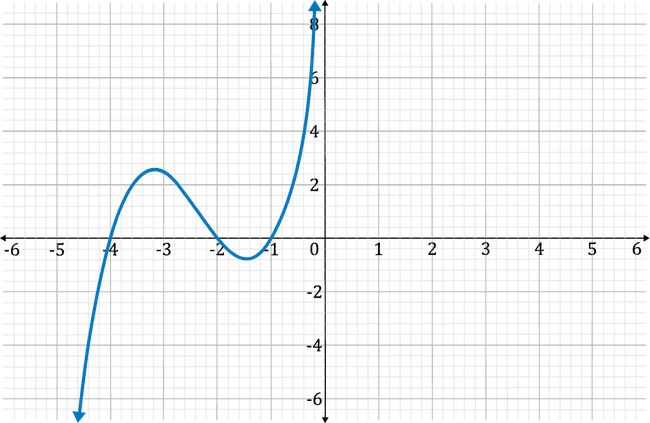
Based on the graph, which of the following statements is true?
The graph of a function is even when it has symmetry about the \(y\)-axis. The graph will appear to be mirrored about the \(y\)-axis. In other words, reflecting the part of the graph of the function that lies to the right of the \(y\)-axis produces the part of the graph that lies on the left side of the \(y\)-axis. This means that any point \((x,y)\) on the graph of the function reflects to \((-x,y)\) which is also on the graph of the function. Below is the graph of the function and its reflection about the \(y\)-axis, shown in red.
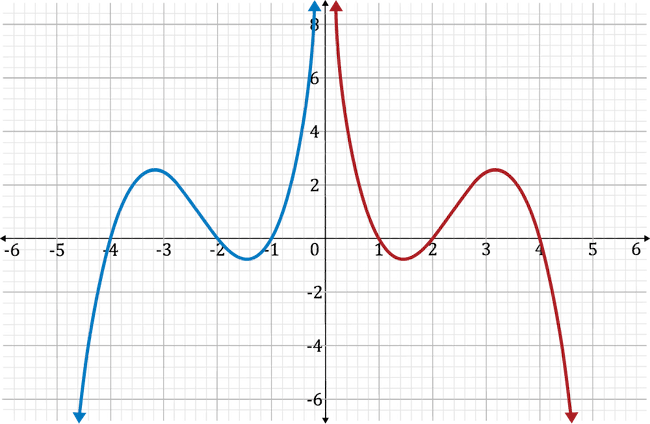
Notice that the reflected graph of \(y=f(x)\) about the \(y\)-axis produces an entirely different graph. Thus, the graph of \(y=f(x)\) does not have symmetry about the \(y\)-axis, so it is not an even function.
The graph of a function is odd when it has symmetry about the origin. The graph will appear to be the same when it is rotated 180° about the origin. This means that any point \((x,y)\) on the graph of the function reflects to \((-x,-y)\) which is also on the graph of the function. Below is a graph of the function and its reflection about the origin shown in red.
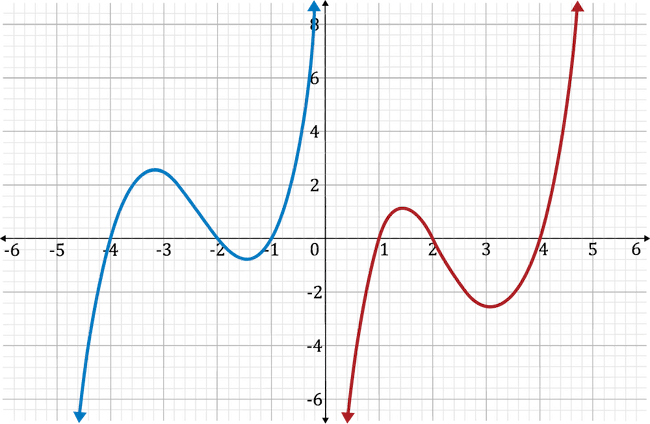
Notice that the reflected graph of \(y=f(x)\) about the origin produces an entirely different graph. Thus, the graph of \(y=f(x)\) does not have symmetry about the origin, so is not an odd function. Additionally, another condition to consider when determining if the graph of a function is odd, is that it must pass through the origin.
Therefore, the graph of \(y=f(x)\) is neither an even nor an odd function.
Which of the following statements best describes the function \(f\left(x\right)=2x-x^3\)?
A function, \(y=f(x)\), is an even function when replacing the \(x\)-value in the function with \(-x\) does not change the value of the function. That is, \(y=f\left(-x\right)=f(x)\). Replacing \(x\) with \(-x\) for our function and simplifying, we get:
Since \(f\left(-x\right)=-f(x)\), the value of the function changes sign when replacing it with \(-x\), so the function is not an even function.
A function, \(y=f(x)\), is an odd function when replacing the \(x\)-value in the function with \(-x\) changes the value of the function. That is, \(y=f\left(-x\right)=-f(x)\). As we saw from above, replacing \(x\) with \(-x\) for our function produces \(f\left(-x\right)=-f(x)\), so our function is an odd function.
Which of the following statements best describes the function \(f\left(x\right)=x^4-2x^2+1\)?
A function, \(y=f(x)\), is an even function when replacing the \(x\)-value in the function with \(-x\) does not change the value of the function. That is, \(y=f\left(-x\right)=f(x)\). Replacing \(x\) with \(-x\) for our function and simplifying, we get:
Since \(f\left(-x\right)=f(x)\), the value of the function does not change sign when replacing it with \(-x\), so the function is an even function.
A function, \(y=f(x)\), is an odd function when replacing the \(x\)-value in the function with \(-x\) changes the value of the function. That is, \(y=f\left(-x\right)=-f(x)\). As we saw from above, replacing \(x\) with \(-x\) for our function produces \(f\left(-x\right)=f(x)\), so our function is not an odd function.
Therefore, the function is only an even function.
A picture of a cross section of a bowl-shaped skateboard ramp that was taken at a skatepark is shown on the coordinate plane below. Let the cross section of the curved shape of the ramp be the function \(y=f(x)\).
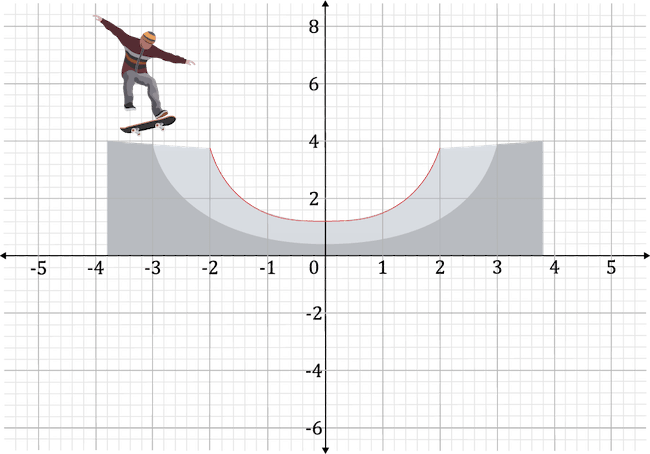
If we are looking at the bottom of the bowl-shaped ramp, which of the following statements appears to be true about the graph of the function that represents the cross section of the ramp?
The graph of a function is even when it has symmetry about the \(y\)-axis. The graph will appear to be mirrored about the \(y\)-axis. Reflecting the part of the graph of the function that lies to the right of the \(y\)-axis produces the part of the graph that lies on the left side of the \(y\)-axis. This means that any point \((x,y)\) on the graph of the function reflects to \((-x,y)\) which is also on the graph of the function. Below is the graph of the ramp and its reflection about the \(y\)-axis.
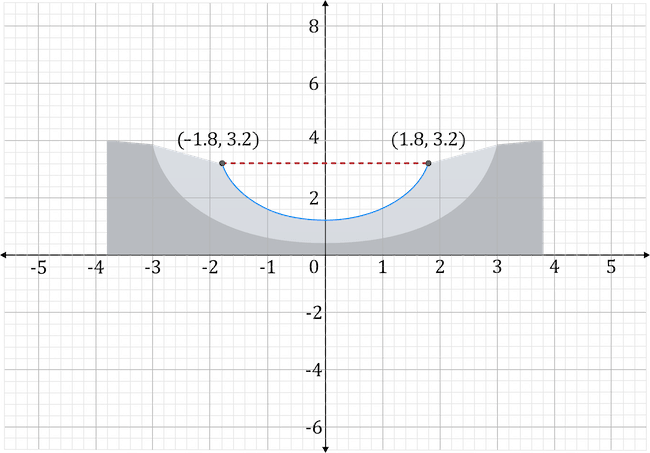
Notice that reflecting the side of the graph to the right of the \(y\)-axis about the \(y\)-axis produces the portion of the ramp that is to the left of the \(y\)-axis. One such point on the graph of the ramp bears this out and this is true for all points on the graph of the ramp. Thus, the graph the function \(y=f(x)\) that represents the skate ramp has symmetry about the \(y\)-axis, so it is an even function.
The graph of a function is odd when it has symmetry about the origin. The graph will appear to be the same when it is rotated 180° about the origin. This means that any point \((x,y)\) on the graph of the function reflects to \((-x,-y)\) which is also on the graph of the function. Below is the graph of the ramp and its reflection about the origin.
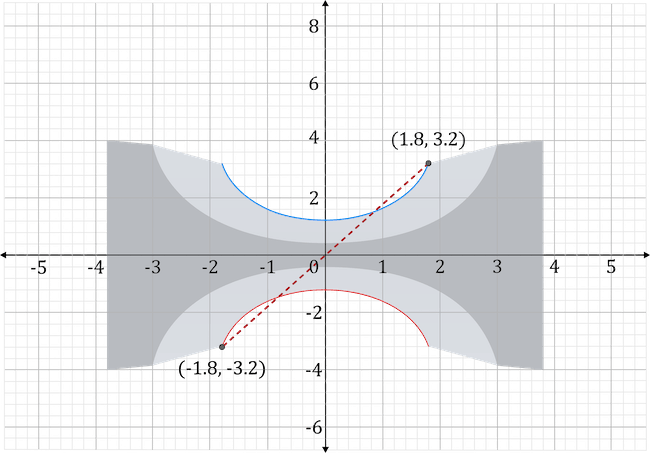
Notice that the reflected graph of the ramp about the origin produces an entirely different graph. Thus, the graph of the ramp does not have symmetry about the origin, so is not an odd function.
Therefore, the graph of \(y=f(x)\) that represents the skate ramp is only an even function.
Radio waves are electromagnetic waves that travel at or close to the speed of light. There are many types of radio waves that occur in nature, such as light waves, and ones that are generated artificially with machines. One such artificial wave, called an FM (frequency modulation) radio wave, transmits a carrier signal from a radio station that carries information to your radio’s antenna in which the amplitude of the carrier signal is constant, but the frequency is modulated, or changes. Below is an example of 2 cycles, or periods, of an FM carrier wave that has been modulated.
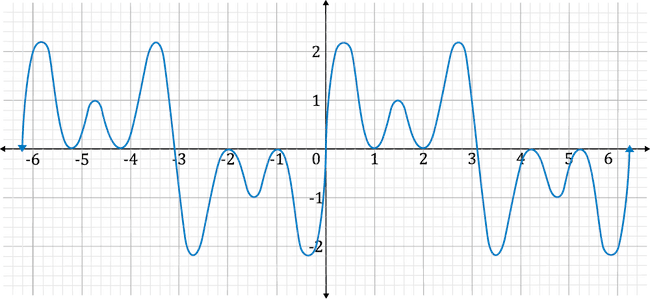
According to the graph of the signal, the FM radio wave is:
The graph of a function is even when it has symmetry about the \(y\)-axis. The graph will appear to be mirrored about the \(y\)-axis. Reflecting the part of the graph of the function that lies to the right of the \(y\)-axis produces the part of the graph that lies on the left side of the \(y\)-axis. This means that any point \((x,y)\) on the graph of the function reflects to \((-x,y)\) which is also on the graph of the function. Below is a graph of the radio wave and its reflection about the \(y\)-axis, shown in red.
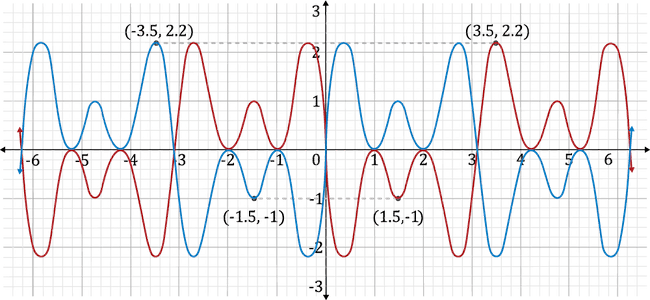
Notice that the reflected graph of the radio wave about the \(y\)-axis produces an entirely different graph. Thus, the graph of the radio wave does not have symmetry about the \(y\)-axis, so is not an even function.
The graph of a function is odd when it has symmetry about the origin. The graph will appear to be the same when it is rotated 180° about the origin. This means that any point \((x,y)\) on the graph of the function reflects to \((-x,-y)\) which is also on the graph of the function. Below is the graph of the radio wave and its reflected graph about the origin.
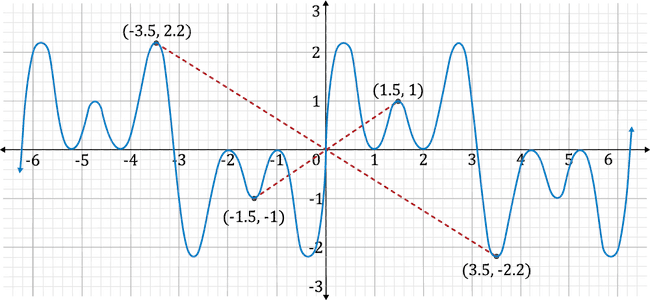
Notice that reflecting the side of the graph to the right of the \(y\)-axis about the origin produces the portion of the ramp that is to the left of the \(y\)-axis. Two such points on the graph of the radio wave bear this out, and this is true for all points on the graph of the radio wave. Thus, the graph of the function that represents the radio wave has symmetry about the origin, so it is an odd function.

