Solving One-Step Equations

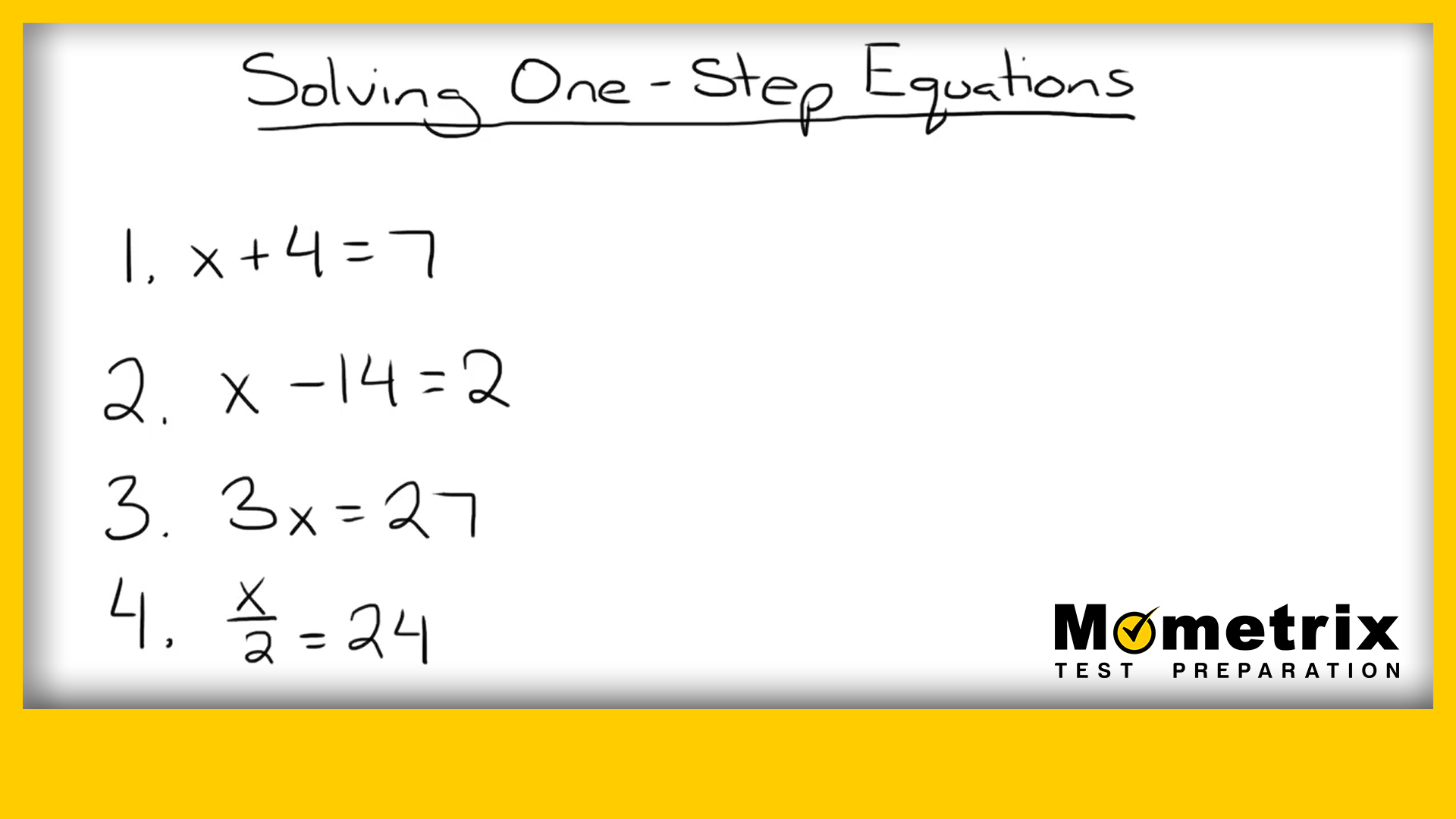
Hello! Today we’re going to take a look at how to solve one-step equations. This is one of the most fundamental tools for solving equations. So, let’s start with an example.

\(x+4=7\)

One thing that’s important to remember is that an equation means that the left side is equal to the right side. So in this case, \(x+4\) is equal to 7. To “solve an equation” means to find the value of \(x\) that makes the equation true. So, if we think about this logically for just a minute, we know that \(x\) has to be equal to 3, because \(3+4=7\).

This works well for simple equations like this, but once we get to more complex equations, it won’t work quite as well. So we’re going to take a look at a process that will work every time for solving equations. So, let’s look back at our equation: \(x+4=7\). To find the value of \(x\), we need to undo the operations around the \(x\) to get \(x\) by itself on one side of the equation. So in this case, we have \(x+4\), and we want to undo that \(+4\). So, since 4 is being added to \(x\), the opposite of addition is subtraction, so we’re going to subtract 4.

\(x+4-4\)

So if we have \(x+4\), and then we subtract 4, we’ll be left with just \(x\).

Now, one thing to pay attention to that’s really important is that an equation means that both sides are balanced — remember, we said the left side is equal to the right side, so our equation, we call this being balanced. Now, if we do something to the left side, we then make it unbalanced because we haven’t done anything to the right side. So, in order to balance this equation, we have to do the exact same thing to the right side. So if we subtract 4 from the left side, we have to subtract 4 from the right side, in order for this still to be true.

\(x+4-4=7-4\)

So, if we subtract \(7-4\), we’ll get 3, so \(x=3\), which is what we said earlier.

To double check our work one last time, we can plug in our value for \(x\) into our original equation. So we had:

\((3)+4=7\)
 
\(7=7\)

\(7=7\) is a true statement, so we know that \(x=3\) is the correct answer.

Now let’s take a look at a problem that involves subtraction:

\(x-14=2\)

So remember, we said we want to undo the operation around the \(x\), so here we’re subtracting 14. The opposite of subtraction is addition, so we’re going to add 14. Remember, our equation must be balanced, meaning that whatever we do to the left side, we also have to do to the right side, so if we add 14 to the left side, we need to add 14 to the right side.

\(x-14+14=2+14\)

Our 14s here will cancel out because we’re subtracting 14 and then adding 14, so that means we won’t have to worry about these. And we are just left with \(x\) on the left side, which is what we’re wanting. And then \(2+14=16\), so:

\(x=16\)

If we want to double check our answer, we can plug in our value for \(x\) back into our original equation, right where we see an \(x\). So if \(x=16\), we have:

\((16)-14=2\)

\(2=2\)

\(2=2\) is a true statement, so that means that our answer, \(x=16\), is correct.

Now let’s move on to multiplication and division. Addition and subtraction are opposite operations of one another, and so are multiplication and division. So, if we have an equation like

\(3x=27\)

this means \(3x=27\). Remember, if you have a number right next to a letter, or variable, that means that they’re being multiplied, even if you don’t see a multiplication symbol in the middle. So this means, \(3x=27\).

We just said that the opposite of multiplication is division, so in order to get rid of this multiplication by 3, we’re going to divide by 3 on both sides.

\(\frac{3x}{3}=\frac{27}{3}\)

Notice that I wrote a little fraction bar right here. Remember that fractions mean divide, so I could have written a division symbol like this (\(\div\)), and that also would have been correct. But fractions always mean divide, so this is just one way to write it. So, now we have \(3\div 3=1\), so we have \(1\cdot x=27\div 3\), which is 9. Now, 1 times anything is that thing, so \(1\cdot x\) is just \(x\). So this gives us:

\(x=9\)

Again, if we want to check our answer, we can plug in \(x\) back into our original equation. So we have:

\(3(9)=27\)
 
\(27=27\)

So we have \(27=27\), which is a true statement. And that tells us that our answer, \(x=9\), is true.

Let’s look at one more example, this time using division.

\(\frac{x}{2}=24\)

Remember, we said earlier that a fraction bar means divide, so this is the same as saying, \(x\div 2=24\), they’re the exact same thing. So, since we have division, and we need to do the opposite operation of division to both sides, we’re going to multiply both sides by 2.

\(2\times \frac{x}{2}=24\times 2\)

If we divide by 2 and then multiply by 2, those will cancel out, and we’ll be left with 1. Because \(x\) divided by 2 and then multiplied by 2 gives us just \(x\), which is \(1x\). So \(1x\), or \(x\), it always means the same thing. And then over here we have, \(24\times 2=48\). So our answer is:

\(x=48\)

But let’s double check just to be sure. Let’s go back to this original equation right here (\(\frac{x}{2}=24\)).

\(\frac{48}{2}=24\)
 
\(24=24\)

\(24=24\), which is a true statement. And that means that, \(x=48\), is the correct answer. So, I hope that this video helped you understand how to solve one-step equations! This is super important for all of the equations that you will be solving in the future. Thanks for watching and happy studying!

One-Step Equation Practice Questions